In the realm of mathematics, the reflexive property stands as a fundamental concept that permeates various mathematical structures. This property dictates that every element within a given set relates to itself in a specific way, offering a crucial foundation for understanding mathematical operations and structures.
Delving deeper into the reflexive property, we explore its definition, applications, and significance in different mathematical contexts, uncovering its profound implications for problem-solving and analysis.
In mathematics, the reflexive property states that for any element in a set, that element is equal to itself. This concept extends beyond abstract mathematical concepts and finds practical applications in various fields, such as real estate. When considering real estate in Saratoga NY , the reflexive property reminds us that every property is inherently equal to itself, providing a foundation for fair and equitable property evaluations.
Reflexive Property
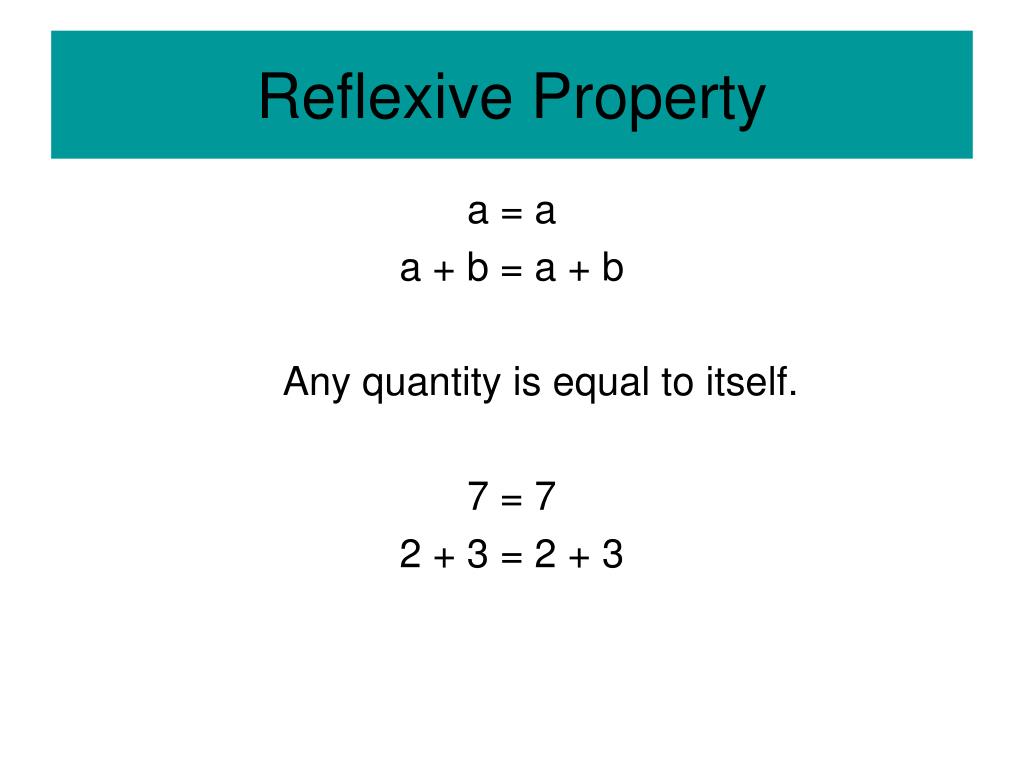
In mathematics, the reflexive property states that every element in a set is related to itself.
This property is formally defined as:
For any set A and any element a in A, aRa.
Reflexive Property in Different Mathematical Structures
The reflexive property applies to various mathematical structures, including:
- Sets:For any set A, the relation “is an element of” is reflexive because every element in A is an element of itself.
- Matrices:The equality relation is reflexive for matrices. For any matrix A, A = A.
- Graphs:The adjacency relation is reflexive for graphs. For any vertex v in a graph, v is adjacent to itself.
Reflexive Property and Symmetry
The reflexive property implies symmetry, meaning that if aRb, then bRa. This is because for any element a in a set A, aRa implies that b = a, and hence aRb implies bRa.
Applications of the Reflexive Property
The reflexive property has numerous applications in various fields, including:
- Computer science:In data structures such as sets and graphs, the reflexive property ensures that every element can be uniquely identified.
- Physics:The equality relation in physics represents the identity of objects, which is a fundamental principle.
- Economics:The reflexive property of the preference relation allows individuals to compare their own preferences consistently.
Illustrative Examples, Reflexive property
| Relation | Domain | Reflexive Elements |
|---|---|---|
| “is equal to” | Set of real numbers | Every real number is equal to itself |
| “is a subset of” | Set of all sets | Every set is a subset of itself |
| “is adjacent to” | Graph with n vertices | Every vertex is adjacent to itself (self-loop) |
Advanced Concepts
In the context of partial orders and equivalence relations, the reflexive property plays a crucial role in defining these structures:
- Partial orders:A partial order is a reflexive, antisymmetric, and transitive relation.
- Equivalence relations:An equivalence relation is a reflexive, symmetric, and transitive relation.
Ending Remarks
In conclusion, the reflexive property serves as a cornerstone of mathematical structures, providing a framework for understanding relations, sets, matrices, graphs, and more. Its presence ensures that every element within a set bears a fundamental relationship with itself, influencing the properties and operations defined within those structures.
By grasping the essence of the reflexive property, mathematicians and individuals alike gain a deeper appreciation for the intricate tapestry of mathematical concepts and their far-reaching applications.
FAQ Summary: Reflexive Property
What is the mathematical definition of the reflexive property?
The reflexive property states that for any element ‘a’ in a set ‘A,’ the relation ‘aRa’ holds true, where ‘R’ represents the relation defined on the set.
How does the reflexive property apply to graphs?
In graph theory, the reflexive property ensures that every vertex in a graph is connected to itself by a loop. This property plays a crucial role in defining the structure and properties of graphs.
What is the relationship between the reflexive property and symmetry?
The reflexive property implies symmetry. If a relation is reflexive, then it must also be symmetric, meaning that if ‘aRb’ holds true, then ‘bRa’ must also hold true.
Can you provide an example of a non-reflexive relation?
The ‘greater than’ relation (>) is an example of a non-reflexive relation. For any number ‘a,’ ‘a>a’ does not hold true.