Commutative property ex, a fundamental property in mathematics, dictates that changing the order of operands in specific operations does not alter the result. This concept plays a crucial role in simplifying calculations and enhancing our understanding of mathematical operations.
Delving into the commutative property ex, we will explore its definition, applications in arithmetic and algebra, real-world examples, and its significance in various mathematical structures. Additionally, we will shed light on non-commutative operations and their applications.
Commutative Property Definition
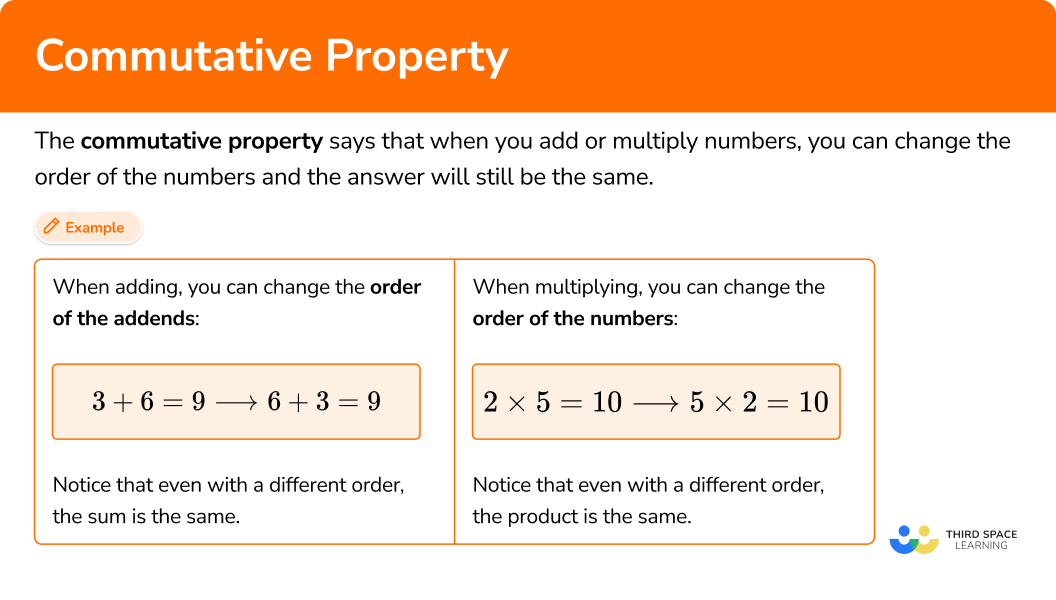
In mathematics, the commutative property states that changing the order of operands in an operation does not change the result. In other words, for any two operands a and b, the operation a ∘ b is equal to b ∘ a.
Common examples of commutative operations include addition, multiplication, and set union. For instance, in addition, the sum of 2 and 3 is the same as the sum of 3 and 2, i.e., 2 + 3 = 3 + 2 = 5. Similarly, in multiplication, the product of 4 and 5 is the same as the product of 5 and 4, i.e.,
4 × 5 = 5 × 4 = 20.
Commutative Property in Arithmetic
The commutative property holds true for both addition and multiplication in arithmetic. This means that when adding or multiplying two numbers, the order of the numbers does not affect the result.
- Addition: a + b = b + a
- Multiplication: a × b = b × a
However, there are exceptions to the commutative property in arithmetic. For example, subtraction and division are not commutative. That is, a – b ≠ b – a and a ÷ b ≠ b ÷ a.
Commutative Property in Algebra
The commutative property also applies to algebraic expressions. For instance, consider the expression 2x + 3y. The commutative property tells us that 2x + 3y is equal to 3y + 2x. This property is particularly useful when simplifying algebraic expressions.
The commutative property for multiplication also extends to algebraic expressions. For example, the expression 2xy is equal to x2y.
Commutative Property in Real-World Applications, Commutative property ex
The commutative property has numerous applications in real-life situations. For instance, in calculating the total cost of items in a grocery store, the order in which the prices are added does not affect the total cost.
Another example is in calculating the area of a rectangle. The area of a rectangle is equal to its length multiplied by its width. Regardless of the order in which the length and width are multiplied, the area remains the same.
Last Recap: Commutative Property Ex
In conclusion, the commutative property ex serves as a cornerstone of mathematical operations, providing a framework for simplifying calculations and fostering a deeper comprehension of mathematical concepts. Its applications extend beyond the realm of mathematics, influencing fields such as physics, engineering, and computer science.
By understanding the nuances of the commutative property ex, we empower ourselves with a valuable tool that enhances our problem-solving abilities and enriches our mathematical journey.
Question Bank
What is the commutative property?
The commutative property of addition and multiplication states that changing the order of the operands does not change the result. This property is fundamental in mathematics and is used extensively in various fields, including real estate. For instance, when calculating the area of a rectangular property, the order in which the length and width are multiplied does not affect the final result.
You can find more information and resources related to real estate logos here . Understanding the commutative property and its applications is essential for accurate calculations and effective problem-solving in real estate and other mathematical domains.
The commutative property states that changing the order of operands in certain operations does not affect the result.
Which operations satisfy the commutative property?
Addition and multiplication are commutative operations, meaning the order of the operands can be changed without altering the result.
Are there any exceptions to the commutative property?
Yes, subtraction and division are not commutative operations. Changing the order of the operands in these operations will change the result.