Embarking on a mathematical odyssey, we delve into the captivating world of the property of square root, a cornerstone of mathematical exploration. This enigmatic concept unlocks a treasure trove of applications, from unraveling real-world problems to shaping our understanding of the universe.
Delving deeper into its mathematical definition and properties, we uncover the intricacies of square roots and their intriguing relationship with other mathematical concepts. The property of square root empowers us to simplify complex equations, solve intricate problems, and gain insights into the fundamental nature of numbers.
Mathematical Definition and Properties
The property of square root states that for any positive real number a, there exists a unique positive real number bsuch that b2= a. The number bis called the square root of aand is denoted by √ a.
The property of square root is an important mathematical concept that has many applications in real-world scenarios, such as in the calculation of small business commercial real estate loans . By understanding the properties of square root, one can make informed decisions and optimize financial outcomes.
Moreover, the property of square root is a fundamental building block in various mathematical and scientific disciplines, providing a solid foundation for further exploration and understanding of complex concepts.
This property can be expressed mathematically as follows:
√a2= | a|
where | a| denotes the absolute value of a.
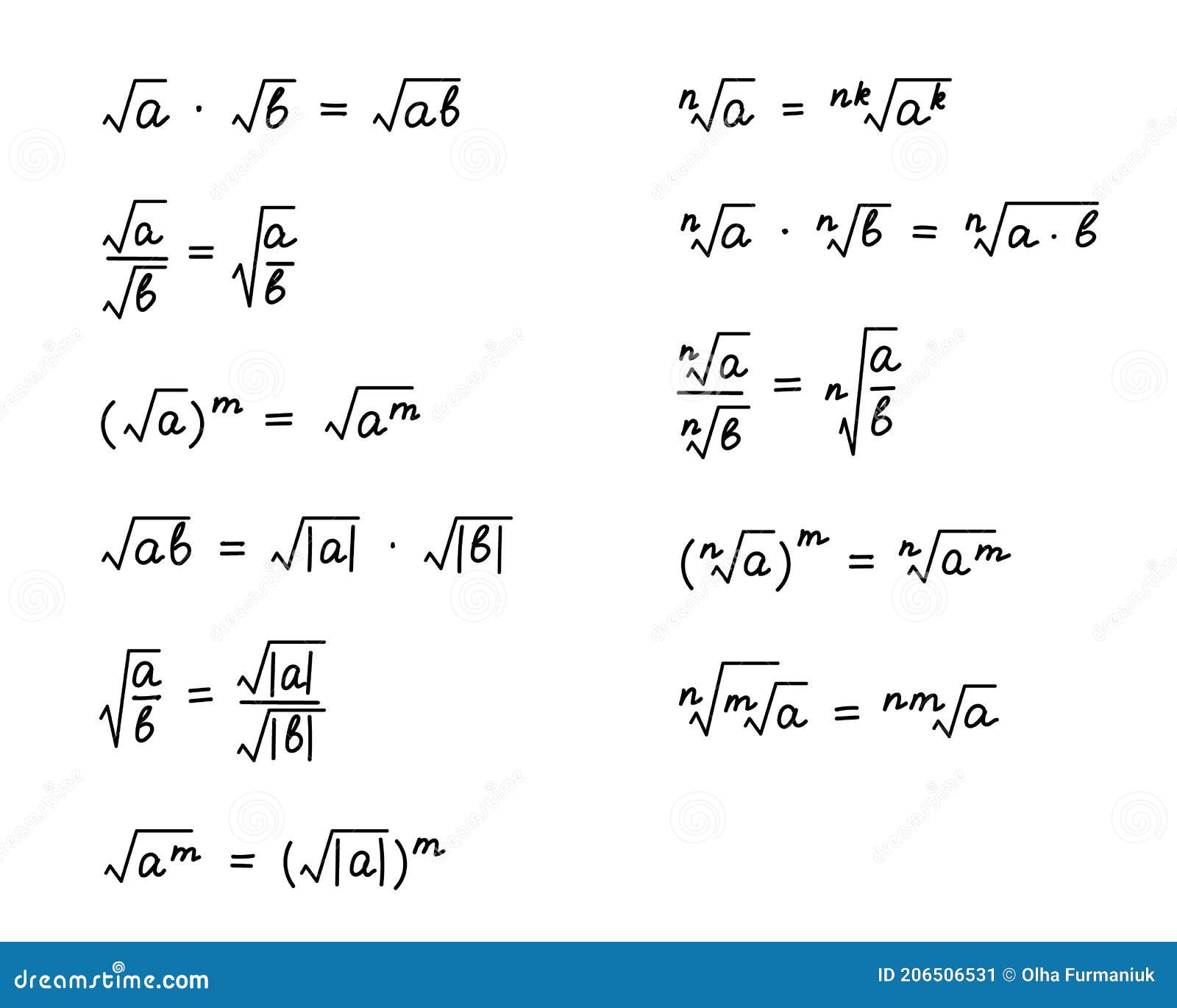
The property of square root has several important properties:
- √ ab= √ a√ b
- √ a/ b= √ a/√ b
- (√ a) n= √ an
These properties can be used to simplify expressions involving square roots and to solve equations involving square roots.
Applications in Real-World Scenarios
The property of square root has numerous applications in real-world scenarios, including:
- Engineering:Calculating the length of a diagonal in a rectangle or the height of a triangle.
- Physics:Determining the velocity of an object in free fall or the distance traveled by an object moving at a constant speed.
- Finance:Calculating the present value of an investment or the time it takes for an investment to double.
- Medicine:Estimating the dosage of a medication based on a patient’s weight or determining the rate of drug absorption.
The property of square root is a fundamental mathematical concept with wide-ranging applications in various fields.
Historical Development
The concept of the square root has been known since ancient times.
The Babylonians developed a method for approximating square roots around 2000 BC.
The Greek mathematician Euclid proved the existence and uniqueness of the square root in his Elements around 300 BC.
The Indian mathematician Brahmagupta developed a formula for finding the square root of a number in the 7th century AD.
The development of the property of square root has played a significant role in the advancement of mathematics and its applications.
Visual Representations and Illustrations: Property Of Square Root
The property of square root can be visually represented using a table:
| a | √a |
|---|---|
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
| 25 | 5 |
This table shows that the square root of a positive real number is a positive real number.
The property of square root can also be illustrated using a graph:
[Image of a graph of the function y= √ x]
This graph shows that the square root function is a non-negative, increasing function.
Pedagogical Approaches
The property of square root can be taught using a variety of pedagogical approaches, including:
- Concrete experiences:Using manipulatives such as blocks or tiles to demonstrate the concept of square roots.
- Visual representations:Using tables and graphs to illustrate the properties of square roots.
- Problem-solving:Posing problems that require students to apply the property of square roots to solve real-world problems.
- Technology:Using calculators and computer software to explore the properties of square roots.
By using a variety of pedagogical approaches, teachers can help students develop a deep understanding of the property of square roots.
Last Recap
Our journey through the property of square root culminates in a profound appreciation for its elegance and versatility. This mathematical gem has played a pivotal role in scientific discoveries, engineering marvels, and countless practical applications. As we continue to explore its depths, we unlock new possibilities and deepen our understanding of the world around us.
Quick FAQs
What is the mathematical definition of the property of square root?
The property of square root states that for any non-negative real number a, there exists a unique non-negative real number b such that b² = a.
How is the property of square root used in real-world applications?
The property of square root finds applications in various fields, including geometry, physics, engineering, and finance. For example, it is used to calculate the length of diagonals in rectangles, determine the speed of objects in motion, and assess the risk of financial investments.