The Zero Product Property is a fundamental mathematical concept that plays a crucial role in solving equations. It states that if the product of two factors is zero, then at least one of the factors must be zero. This property has wide-ranging applications in various fields, including algebra, geometry, and physics.
In this comprehensive guide, we will delve into the Zero Product Property, exploring its definition, applications, and limitations. We will also provide step-by-step procedures for solving equations using this property, along with real-world examples and FAQs to enhance understanding.
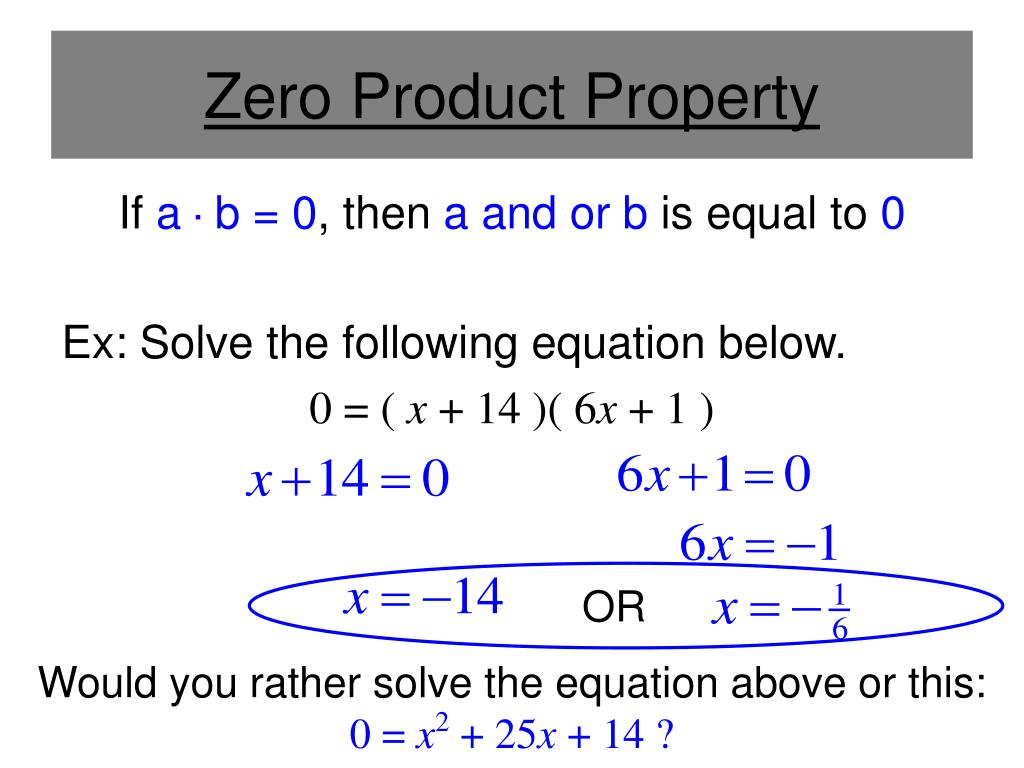
Zero Product Property
The Zero Product Property states that if the product of two or more numbers is zero, then at least one of the numbers must be zero.
Solving Equations Using Zero Product Property
To solve equations using the Zero Product Property, set each factor equal to zero and solve for the variable.
Applications of Zero Product Property
The Zero Product Property has applications in various fields, including:
- Solving equations in mathematics
- Simplifying algebraic expressions
- Finding roots of polynomials
- Modeling real-world situations
Limitations of Zero Product Property
The Zero Product Property does not apply to:
- Equations with complex numbers
- Equations with exponents
- Equations with radicals
Examples of Zero Product Property
| Equation | Property Used | Solution |
|---|---|---|
(x
|
Zero Product Property | x = 2 or x =
|
(y + 5)(y
|
Zero Product Property | y =
|
Methods for Solving Equations Using Zero Product Property
- Set each factor equal to zero.
- Solve each equation for the variable.
- Combine the solutions.
Procedures for Solving Equations Using Zero Product Property
- Set each factor equal to zero.
- Solve each equation for the variable.
- Combine the solutions.
Illustrations of Zero Product Property
The Zero Product Property can be illustrated using a Venn diagram.
If the product of two sets is empty, then at least one of the sets must be empty.

Concluding Remarks
In conclusion, the Zero Product Property is a powerful tool for solving equations. By understanding its concept and limitations, we can effectively apply it to a wide range of mathematical problems. This guide has provided a comprehensive overview of the property, equipping readers with the knowledge and skills to utilize it with confidence.
Commonly Asked Questions: Zero Product Property
What is the Zero Product Property?
The zero product property states that if the product of two numbers is zero, then at least one of the numbers must be zero. This property can be applied to a variety of mathematical problems, including finding the solutions to equations and simplifying expressions.
In the context of commercial real estate loans houston , the zero product property can be used to determine whether a loan is eligible for a particular program or to calculate the amount of interest that will be charged. Understanding the zero product property is essential for anyone who works with mathematics or finance.
The Zero Product Property states that if the product of two factors is zero, then at least one of the factors must be zero.
How can I use the Zero Product Property to solve equations?
To solve equations using the Zero Product Property, set the expression equal to zero and then factor it. If any factor is zero, then the corresponding variable is the solution.
What are some limitations of the Zero Product Property?
The Zero Product Property does not apply to equations with complex numbers or equations that are not factorable.